AWOKK, Day 5: Harold Reiter’s Puzzles

You’ve made it! Today is Day 5 in MJ4MF’s A Week of KenKen series. In case you missed the fun we’ve had previously…

• Day 1: Introduction
• Day 2: The KENtathlon
• Day 3: KenKen Times
• Day 4: My KenKen Puzzles

Like Thomas Snyder, Harold Reiter believes that human-generated KenKen puzzles are superior to those created by computer. But Harold pushes the envelope by developing variations on KenKen that include Primal KenKen, 3-D KenKen, n × n KenKen puzzles that use numbers other than 1‑n, and Clueless KenKen — which contain no clues and, contrary to popular belief, were not invented by Cher Horowitz. Recently, in fact, he sent me a No-Op KenKen puzzle that used the floor function.

The following standard KenKen puzzle is one of Harold’s “Big Letter” puzzles. One of the cages forms a capital E, and he made this puzzle for a friend whose name began with — wait for it — the letter E.

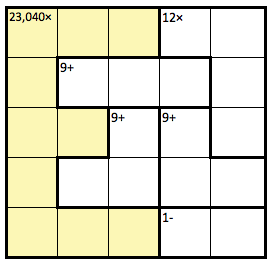No-Op KenKen Puzzles have target numbers in the cages but no operations. All the typical rules of KenKen apply, but it’ll take a little more thought to determine which operation is needed in each cage. The following is Harold’s “No-Op 12” puzzle.

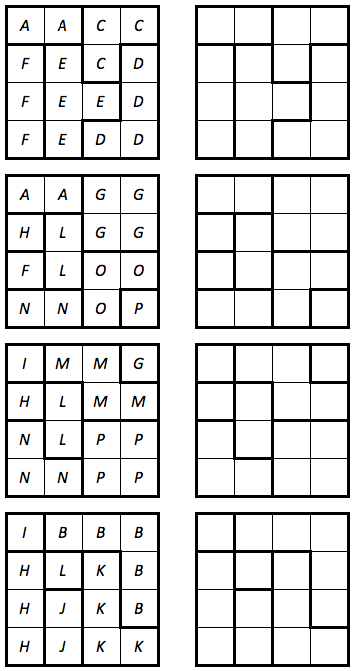
And the piece de resistance of Harold’s creations — though he says the idea for this one actually came from his daughter, Ashley — is the following 3‑D KenKen. Here are Harold’s instructions for this puzzle:

In the puzzle below, there are 48 lines. Each must contain exactly one of the digits 1, 2, 3, and 4. The 16 cages are identified by letters. Each cage is either additive or multiplicative, and the target number for every cage is 12 — meaning that the numbers in the cells of every cage either add or multiply to give 12. Ken you solve it?

This one might need a little more explanation. The four layers of the puzzle work together; for instance, the two A’s on the top layer and the two A’s on the second layer form a four-number cage with a target number of 12. Similarly, the four G’s on the second layer and the lone G in the third layer form a five-number cage with a target number of 12. All standard KenKen rules apply and are extended to three dimensions — no repeat of a digit in any row, column, or tube vector.

I prefer this presentation of the puzzle, which looks like a 3‑D tic-tac-toe board:

But Harold’s presentation of it gives you a blank version side-by-side with the original, so you have a place to record your work:

The KenKen world is indebted to Harold for creating interesting puzzles and for sharing them with a new generation of students at his local math events.

For more of Harold’s puzzles, and for suggestions on how to create your own, check out KenKen, Thinking Globally.

Wee Wee Can-Can Doodoo KenKen

My sons love words with repeating syllables, such as…

• pee pee
• poo poo
• knock knock
• bam bam
• Pop Pop
• couscous

But, by far, their favorite word that exhibits exact reduplication is KenKen.

My friend Harold Reiter creates KenKen puzzles. His aren’t the garden-variety, generated-by-computer-algorithm type, though. His puzzles have interesting features, such as including the first initial of his name as one of the cages:

Note that the puzzle above also has a “clueless cage” in the upper right corner; to solve the puzzle, it is not necessary to know the value or operation associated with this three-square cage. (If KenKen is new to you, check out the rules for solving.)

On a recent rainy afternoon, my twin four-year-old sons Alex and Eli solved the puzzle above. On their own, they identified the set of seven numbers that could uniquely fill the H. Eli said, “I know that 8 × 6 = 48, and 8 = 4 × 2 and 6 = 3 × 2, so it’s {1, 1, 1, 2, 2, 3, 4}.” I asked if there were any other possibilities. Eli continued, “Well, 1 × 1 × 2 × 2 × 2 × 2 × 3 = 48, too, but there can’t be four 2’s, because there are only three columns in the H.” I then had to help with some of the logic about how to place those seven numbers… but once they realized that the center of the H had to be a 1, they were off to the races.

After completing the puzzle, I told Alex and Eli that my friend Harold had created the puzzle. Upon hearing this, Alex turned the paper over. “Let’s make a KenKen puzzle,” he suggested. And why not? I drew a 4 × 4 grid for them, and they proceeded to tell me where to place the digits to create a Latin square. Then, they indicated the location of the cages, as well as the values and operations to use within the cages. To prove that the solution was unique, I created a blank version of the puzzle on a different sheet of paper. When I asked the boys to solve it with me, Alex grabbed the paper on which we had originally designed the puzzle and showed me the solution. (Okay, so maybe they don’t yet understand the concept of uniqueness, but I appreciated Alex’s application of a fundamental problem-solving strategy: reduce it to a previously solved puzzle!)

For your enjoyment, I present Alex and Eli’s first-ever KenKen puzzle.

If you roll your mouse over the puzzle above, you’ll notice the alternate text reads, “Alex and Eli’s KenKen Puzzle for Grandma.” Upon completing the puzzle, the boys suggested that we send the puzzle to their grandmother. So we did. That was over a week ago… and we still haven’t heard a response.

Presentation at Central Connecticut State University

Yesterday morning, I presented a math and technology workshop at Central Connecticut State University. The attendees were teachers who are testing a new Algebra I curriculum. As part of the workshop, the attendees participated in my favorite online activity, and they attempted my favorite problem.

To ensure that I provided appropriate activities, I reviewed a draft of the new curriculum ahead of time. The vocabulary list for the first unit contained the word hendecagon. Guessing that most attendees were unfamiliar with the term, I provided a diagram:

Seriously, a hendecagon is an 11‑sided polygon. The Susan B. Anthony dollar coin is probably the most common example of a hendecagon in everyday life. (As it turns out, there is no connection between the number 11 and Susan B. Anthony, but the reverse side of the coin contains an image adapted from the Apollo 11 mission insignia.)

(For the record, I’d be interested in creating more silly images like the one of the hendecagon above. Are there other math terms that lend themselves to similarly silly drawings? If you think of one, post a comment.)

At the end of the workshop, I gave away several copies of Math Jokes 4 Mathy Folks. To determine who would get the copies, we played my favorite game:

• On a piece of paper, write down a positive integer.
• Share your number with a neighbor (for verification later, if necessary).
• The winner is the person who wrote down the smallest integer not written by anyone else.

The rule for determining a winner often confuses folks, so let me give an example. Let’s say 20 people chose the following numbers:

4, 1, 7, 11, 4, 6, 5, 2, 1, 3, 2, 1, 8, 1, 4, 7, 9, 2, 3, 18

• The smallest integer in the list is 1, but it was written by four people. Hence, none of them would win.
• The next smallest integer is 2, but it was written by three people, so none of them would win, either.
• The number 3 was chosen by two people, and the number 4 was chosen by three people — no winners.
• Which brings us to the number 5. The person who chose 5 is the winner, because she wrote the smallest integer not written by anyone else.
• Although the folks who wrote 6, 8, 9, 11, and 18 wrote numbers that weren’t written by anyone else, they did not choose the smallest numbers not written by anyone else.

As with many things, this game may not sound very fun with a text explanation. But play it a few times. Or better yet — play it the next time you go to dinner with a large group of friends, and the losers have to buy dinner for the winner.

I learned this game from my friend Richard Rusczyk, who referred to it as “Harold’s Game,” because he learned it from our friend Harold Reiter. Despite the name Richard has given the game, I do not believe that Harold is the creator.